Research News

Abstract:

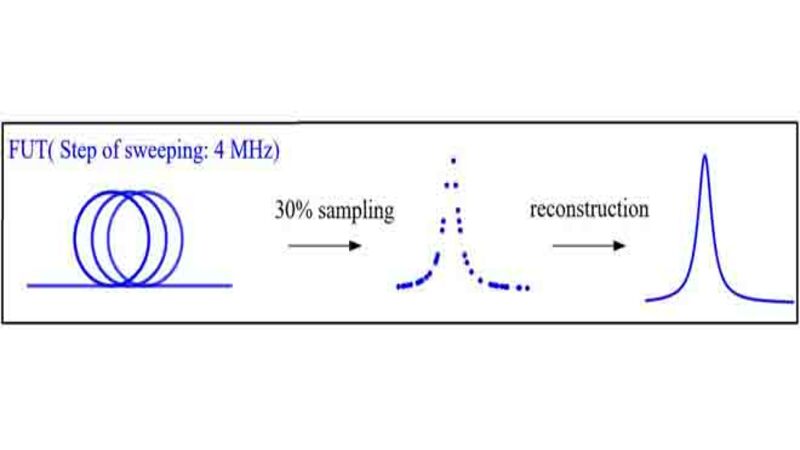
The continuous operation of Brillouin optical fiber distributed sensors requires the acquisition and processing of large amount of data that imposes ample pressure on the data acquisition systems and storage devices. To overcome such limitations, the compressed sensing (CS) method based on K-Singular value decomposition (K-SVD) algorithm as transform is proposed in this paper for the accurate extraction of sensing information with less data acquired from Brillouin optical fiber distributed sensors without the hardware modification. In such algorithm, the parameters of the K-SVD dictionary are selected first through simulation and the signals are sampled and reconstructed successfully. Then, we apply the regularized orthogonal matching pursuit (ROMP) reconstruction algorithm to effectively reconstruct the experimental data obtained with different sampling rates, frequency steps and test temperatures. We also compare the performances of the CS method based on K-SVD with that based on discrete cosine transform (DCT), discrete Fourier transform (DFT) and principle component analysis (PCA) as transforms. The experimental results show that the proposed method can effectively reconstruct the experimental signals with much reduced amount of data as compared to other methods. The proposed method also provides satisfactory measurement accuracy in extracting sensing information. Therefore, the K-SVD based CS method can be an attractive alternative to make Brillouin optical fiber distributed sensors more suitable for faster operation.

Link: https://doi.org/10.1109/JSEN.2022.3191336